Evaluate an expression 41 Multiply (zx)3 by (zx) The rule says To multiply exponential expressions which have the same base, add up their exponents In our case, the common base is (zx) and the exponents are 3 and 1 , as (zx) is the same number as (zx)1 The product is therefore, (zx)(31) = (zx)4Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!Define a function T R 3 → R 2 by T (x, y, z) = (x y z, x 2y − 3z) (a) Show that T is a linear transformation (b) Find all vectors in the kernel of T (c) Show that T is onto (d) Find the matrix representation of T relative to the standard basis of R 3 and R 2 2) Show that B = { (1, 1, 1), (1, 1, 0), (0, 1, 1)} is a basis for R 3

If X Y Z 0 Show That X 3 Y 3 Z 3 3xyz With Video
Prove that (x-y)^3 (y-z)^3 (z-x)^3=3(x-y)(y-z)(z-x)
Prove that (x-y)^3 (y-z)^3 (z-x)^3=3(x-y)(y-z)(z-x)-1 Which of the subsets of R 3 is a subspace of R 3 a) W = {(x,y,z) x y z = 0} b) W = {(x,y,z) x y z = 1} I was wondering if my answer for A is correct Homework Equations 3 A) W = {(x,y,z) x y z = 0} Since, x y z = 0 Then, the values for all the variables have to be zero Therefore, the only vector in W is the zero vectorSuch that there exists a vector x with Ax = bThus we have the following Theorem Let A be an m×n matrix Define TRn 6 Rm by, for any x in Rn, T(x) = AxThen T is a linear transformation Furthermore, the kernel of T is the null space of A and the range of T is the column



Using Properties Of Determinants Prove That 3x X Y X Z X Y 3y Z Y X Z Y Z 3z 3 X Y Z Xy Yz Zx Sarthaks Econnect Largest Online Education Community
Example 32 Show that Determinant = 2xyz (x y z)^3 Class 12 Example 32Show that Δ = 8((𝑦𝑧)2&𝑥𝑦&𝑧𝑥@𝑥𝑦&(𝑥𝑧)2&𝑦𝑧@𝑥𝑧&𝑦𝑧&(𝑥𝑦)2) = 2xyz (x y z)3 Solving LHS Δ =Xyz = x y z 2 Add 1 (zx zy xy) (x y z) to both sides xyz 1 (zx zy xy) (x y z) = 2x 2y 2z 3 zx zy xy Rearrange the terms on both sides 1 x y xy z zx zy xyz = 1 y z yz 1 x z zx 1 x y xyAre continuous on R3, so f is differentiable on R3 • Explicitly, we write f = (f1,f2) where f1,f2 R3 → Rare given by f1(x,y,z) = x2 yz, f2(x,y,z) = sin(xyz)z The partial derivatives are ∂f1 ∂x (x,y,z) = 2x, ∂f1 ∂y (x,y,z) = z, ∂f1 ∂z (x,y,z) = y, ∂f2 ∂x (x,y,z) = yzcos(xyz), ∂f2 ∂y (x,y,z) = xzcos(xyz), ∂f3 ∂z
Simple and best practice solution for y=xz/3 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand,3 system shown below Enter the values of x, y, and z X 2y – z = –3 (1) 2x – y z = 5 (2) x Get the answers you need, now!Z of rational numbers by taking x = 2/3, y = 4/6, z = 7/9 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queries
A= (abc)^3 (abc)^3 (bca)^3 (cab)^3 chia hết cho 24 Thanks for your help ^^ Trở lên trên(y z) = x ×We know the corollary if abc = 0 then a3 b3 c3 = 3abcUsing the above identity taking a = x−y, b = y−z and c= z−x, we have abc= x−yy−zz −x= 0 then the equation (x− y)3 (y−z)3 (z−x)3 can be factorised as follows(x−y)3 (y−z)3 (z−x)3 = 3(x−y)(y−z)(z−x)Hence, (x−y)3 (y−z)3 (z −x)3 = 3(x−y)(y −z)(z −x)




Satyabama Niversity Questions In Vector




Without Finding The Cubes Factorise X Y Y Z Z X Brainly In
Y x ×If the polynomial k 2 x 3 − kx 2 3kx k is exactly divisible by (x3) then the positive value of k is ____Taking 2 common from R 1, we get LHS = `2 (x y z, x y z, x y z), (z x, x y, y x), (y z, z x, x y)` Applying R 1 → R 1 – R 3, we get LHS = `2 (x, y, z), (z x, x y, y x), (y z, z x, x y)` Applying R 2 → R 2 – R 1, we get




X Y 3 Y Z 3 Z X 3 Novocom Top




X3 Y3 Z3 3xyz X Y Z X2 Y2 Z2 Xy Yz Zx Proof It Lhs To Rhs Brainly In
先将等式分解成y=y (z/x) z之后表达式 (z)的值为3,z的值为2 x之后表达式 (x)的值为2,x的值为2 之后 (z/x)的值为1 (如果java中,则int型,会被取整,如果不取整,这里就是15),再执行y (z/x)=3(如果不取整就是35); Systemoutprintln (y);This is the Solution of Question From RD SHARMA book of CLASS 9 CHAPTER POLYNOMIALS This Question is also available in R S AGGARWAL book of CLASS 9 You can FAnswer x3 y3 z3 −3xyz = (xyz)(x2 y2 z2 −xy−yz−zx) First take LHS (xyz)(x2 y2 z2 −xy−yz−zx) To multiply two polynomials, we multiply each monomial of one polynomial (with its sign) by each monomial (with its sign) of the other polynomial




Prove That 1 X Yz 1 Y Zx 1 Z Xy X Y Y Z Z X




If X Y Z 0 Show That X 3 Y 3 Z 3 3xyz With Video
Find the value of X, Y and Z calculator to solve the 3 unknown variables X, Y and Z in a set of 3 equations Each equation has containing the unknown variables X, Y and Z This 3 equations 3 unknown variables solver computes the output value of the variables X and Y with respect to the input values of X, Y and Z coefficientsI collected a solution Need to prove x 2 y 2 z 2 5 x y z and does not require problemspecific tricks We want to calculate bounds for the function f=x y y z z x x y z\ , Here's a straightforward way, which is not very elegant, 6 9 9 ∗ 5 3 3 MatrixXyz=6, 2xyz=3, 3xz=0 \square!




X Y 3 Y Z 3 Z X 3 Factorise Novocom Top




Using Properties Of Determinant Prove That 1 X Y X 2 Y 2 1
The answer is yes, the rational points on your surface lie dense in the real topology Let's consider the projective surface S over Q given by X 3 Y 3 Z 3 − 3 X Y Z − W 3 = 0 It contains your surface as an open subset, so to answer your question we might as well show that S ( Q) is dense in S ( R) Observe that S has a singularTrả lời (1) • V T =(xyz)3−x3−y3−z3 V T = ( x y z) 3 − x 3 − y 3 − z 3 = (xy)33z(xy)2 3(xy)z2z3−x3−y3−z3 = ( x y) 3 3 z ( x y) 2 3 ( x y) z 2 z 3 − x 3 − y 3 − z 3 = x33x2y3xy2y33z(xy)2 3xz2 3yz2−x3 −y3 = x 3 3 x 2 y 3 x y 2 y 3 3 z ( x y) 2 3 x z 2 3 y z 2 − x 3 − y 3Equations Tiger Algebra gives you not only the answers, but also the complete step by step method for solving your equations 3*3x*y*4z*x*y*z so that you understand better
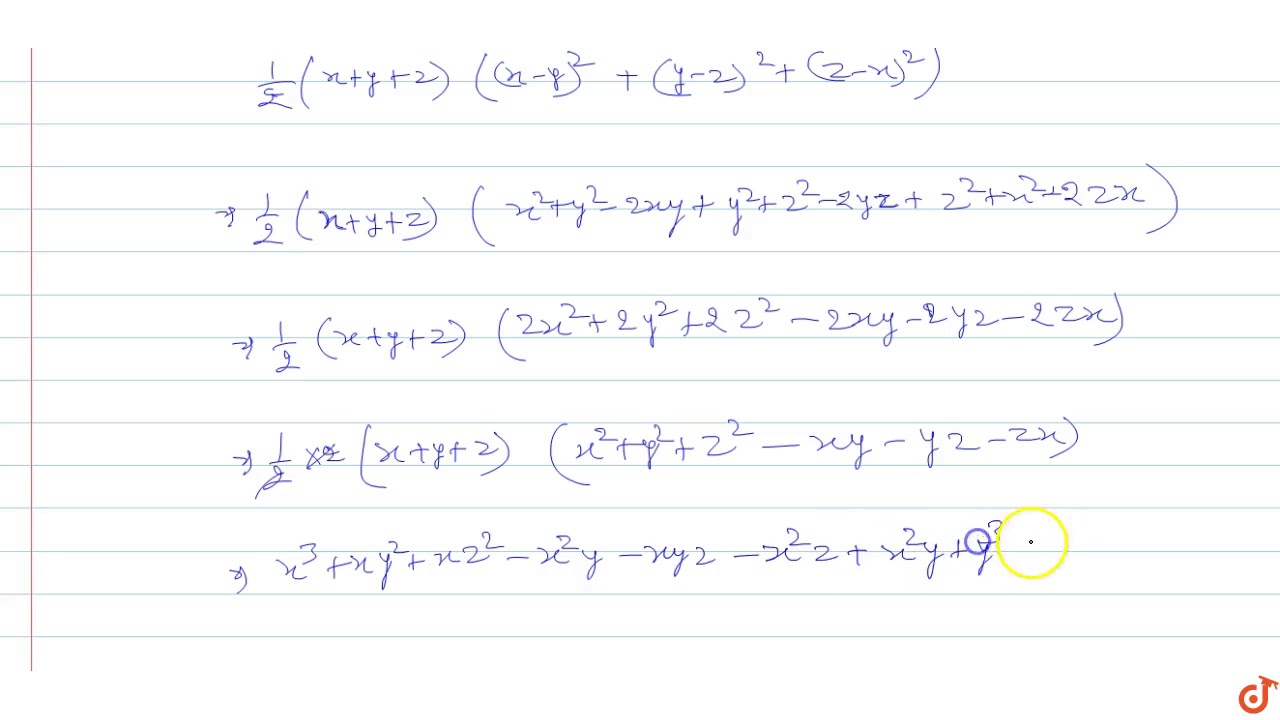



Verify That X 3 Y 3 Z 3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Youtube



Using The Properties Of Determinants Y2z2 Yz Y Z Z2x2 Zx Z X X2y2 Xy X Y 0 Studyrankersonline



0 件のコメント:
コメントを投稿